Solving spherical triangles Edit The general spherical triangle is fully determined by three of its six characteristics (3 sides and 3 angles). Note that the sides a, b, c of a spherical triangle are measured by angular rather than linear units, based on the corresponding central angles. The solution of triangles for non-Euclidean spherical geometry has some differences from the planar case. For example, the sum of the three angles α + β + γ depends on the triangle. In addition, there are no unequal similar triangles, and so the problem of constructing a triangle with specified three angles has a unique solution. The basic relations used to solve a problem are similar to those of the planar case: see Law of cosines (spherical) and Law of sines (spherical). Among other relationships that may be useful are the half-side formula and Napier’s analogies:[7] {displaystyle tan {frac {c}{2}}cos {frac {alpha -beta }{2}}=tan {frac {a+b}{2}}cos {frac {alpha +beta }{2}}} {displaystyle tan {frac {c}{2}}sin {frac {alpha -beta }{2}}=tan {frac {a-b}{2}}sin {frac {alpha +beta }{2}}} {displaystyle cot {frac {gamma }{2}}cos {frac {a-b}{2}}=tan {frac {alpha +beta }{2}}cos {frac {a+b}{2}}} {displaystyle cot {frac {gamma }{2}}sin {frac {a-b}{2}}=tan {frac {alpha -beta }{2}}sin {frac {a+b}{2}}.}  Three sides given Three sides given (spherical SSS) Edit Known: the sides a, b, c (in angular units). The triangle’s angles are computed from the spherical law of cosines: {displaystyle alpha =arccos left({frac {cos a-cos b cos c}{sin b sin c}}right),} {displaystyle beta =arccos left({frac {cos b-cos c cos a}{sin c sin a}}right),} {displaystyle gamma =arccos left({frac {cos c-cos a cos b}{sin a sin b}}right).}  Two sides and the included angle given Two sides and the included angle given (spherical SAS) Edit Known: the sides a, b and the angle γ between them. The side c can be found from the law of cosines: {displaystyle c=arccos left(cos acos b+sin asin bcos gamma right).} The angles α’, β can be calculated as above, or by using Napier’s analogies: {displaystyle alpha =arctan {frac {2sin a}{tan({frac {gamma }{2}})sin(b+a)+cot({frac {gamma }{2}})sin(b-a)}},} {displaystyle beta =arctan {frac {2sin b}{tan({frac {gamma }{2}})sin(a+b)+cot({frac {gamma }{2}})sin(a-b)}}.} This problem arises in the navigation problem of finding the great circle between two points on the earth specified by their latitude and longitude; in this application, it is important to use formulas which are not susceptible to round-off errors. For this purpose, the following formulas (which may be derived using vector algebra) can be used: {displaystyle {begin{aligned}c&=arctan {frac {sqrt {(sin acos b-cos asin bcos gamma )^{2}+(sin bsin gamma )^{2}}}{cos acos b+sin asin bcos gamma }},\alpha &=arctan {frac {sin asin gamma }{sin bcos a-cos bsin acos gamma }},\beta &=arctan {frac {sin bsin gamma }{sin acos b-cos asin bcos gamma }},end{aligned}}} where the signs of the numerators and denominators in these expressions should be used to determine the quadrant of the arctangent.  Two sides and a non-included angle given Two sides and non-included angle given (spherical SSA) Edit This problem is not solvable in all cases; a solution is guaranteed to be unique only if the side length adjacent to the angle is shorter than the other side length. Known: the sides b, c and the angle β not between them. A solution exists if the following condition holds: {displaystyle b>arcsin(sin c,sin beta ).} The angle γ can be found from the spherical law of sines: {displaystyle gamma =arcsin left({frac {sin c,sin beta }{sin b}}right).} As for the plane case, if b < c then there are two solutions: γ and 180° – γ. We can find other characteristics by using Napier’s analogies: {displaystyle a=2arctan left[tan left({tfrac {1}{2}}(b-c)right){frac {sin left({tfrac {1}{2}}(beta +gamma )right)}{sin left({tfrac {1}{2}}(beta -gamma )right)}}right],} {displaystyle alpha =2operatorname {arccot} left[tan left({tfrac {1}{2}}(beta -gamma )right){frac {sin left({tfrac {1}{2}}(b+c)right)}{sin left({tfrac {1}{2}}(b-c)right)}}right].}  One side and two adjacent angles given A side and two adjacent angles given (spherical ASA) Edit
Three angles given (spherical AAA)Edit
Three angles given (spherical AAA)Edit
Known: the angles α, β, γ. From the law of cosines we infer:
{displaystyle a=arccos left({frac {cos alpha +cos beta cos gamma }{sin beta sin gamma }}right),}
{displaystyle b=arccos left({frac {cos beta +cos gamma cos alpha }{sin gamma sin alpha }}right),}
{displaystyle c=arccos left({frac {cos gamma +cos alpha cos beta }{sin alpha sin beta }}right).}
{displaystyle b=arccos left({frac {cos beta +cos gamma cos alpha }{sin gamma sin alpha }}right),}{displaystyle c=arccos left({frac {cos gamma +cos alpha cos beta }{sin alpha sin beta }}right).}
Solving right-angled spherical trianglesEdit
The above algorithms become much simpler if one of the angles of a triangle (for example, the angle C) is the right angle. Such a spherical triangle is fully defined by its two elements, and the other three can be calculated usingNapier’s Pentagon or the following relations.
{displaystyle sin a=sin ccdot sin A}
(from the Law of sines (spherical)){displaystyle tan a=sin bcdot tan A}
{displaystyle cos c=cos acdot cos b}
(from the law of cosines (spherical)){displaystyle tan b=tan ccdot cos A}
{displaystyle cos A=cos acdot sin B}
(also from the law of cosines){displaystyle cos c=cot Acdot cot B}
(from the Law of sines (spherical)){displaystyle tan a=sin bcdot tan A}{displaystyle cos c=cos acdot cos b} (from the law of cosines (spherical)){displaystyle tan b=tan ccdot cos A}{displaystyle cos A=cos acdot sin B} (also from the law of cosines){displaystyle cos c=cot Acdot cot B}
Some applicationsEdit
TriangulationEdit
Distance measurement by triangulation
Main article: Triangulation
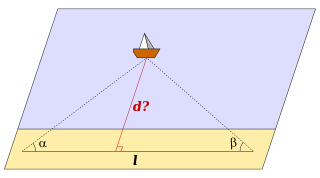
If one wants to measure the distance dfrom shore to a remote ship via triangulation, one marks on the shore two points with known distance lbetween them (the baseline). Let α, β be the angles between the baseline and the direction to the ship.
From the formulae above (ASA case) one can define the length of the triangle height:
{displaystyle d={frac {sin alpha ,sin beta }{sin(alpha +beta )}},l={frac {tan alpha ,tan beta }{tan alpha +tan beta }},l.}
This method is used in cabotage. The angles α, β are defined by observation of familiar landmarks from the ship.
How to measure a mountain’s height
As another example, if one wants to measure the height h of a mountain or a high building, the angles α, β from two ground points to the top are specified. Let l be the distance between these points. From the same ASA case formulas we obtain:
{displaystyle h={frac {sin alpha ,sin beta }{sin(beta -alpha )}},l={frac {tan alpha ,tan beta }{tan beta -tan alpha }},l.}
The distance between two points on the globeEdit
Main article: Great-circle distance
To calculate the distance between two points on the globe,
Point A: latitude λA, longitude LA, andPoint B: latitude λB, longitude LB
we consider the spherical triangle ABC, where C is the North Pole. Some characteristics are:
{displaystyle a=90^{mathrm {o} }-lambda _{mathrm {B} },,}
{displaystyle b=90^{mathrm {o} }-lambda _{mathrm {A} },,}
{displaystyle gamma =L_{mathrm {A} }-L_{mathrm {B} }.,}
{displaystyle b=90^{mathrm {o} }-lambda _{mathrm {A} },,}{displaystyle gamma =L_{mathrm {A} }-L_{mathrm {B} }.,}
If two sides and the included angle given, we obtain from the formulas
{displaystyle mathrm {AB} =Rarccos left[sin lambda _{mathrm {A} },sin lambda _{mathrm {B} }+cos lambda _{mathrm {A} },cos lambda _{mathrm {B} },cos left(L_{mathrm {A} }-L_{mathrm {B} }right)right].}
Here R is the Earth’s radius.
google-site-verification=2j8Us0psP3GGjD12h0fH6_Z08_sRyWQjNbWcaMV4E9A